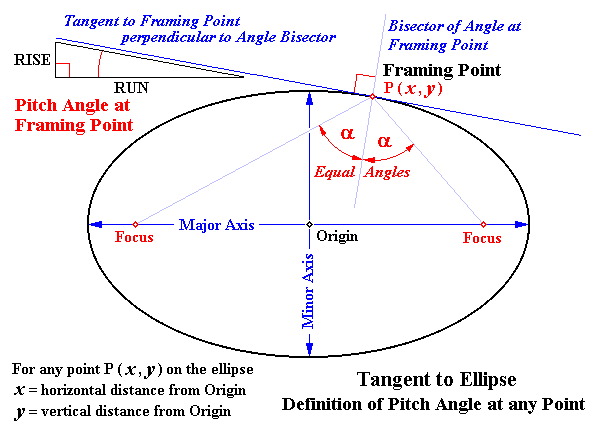
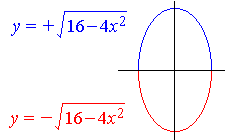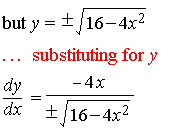Slope of Ellipse at any Point

Geometric Solution of Slope of Ellipse
Tangent to Ellipse at Framing Point
Differentiating with respect to the Eccentric Angle
Parametric Equations of Ellipse with respect to the Eccentric Angle
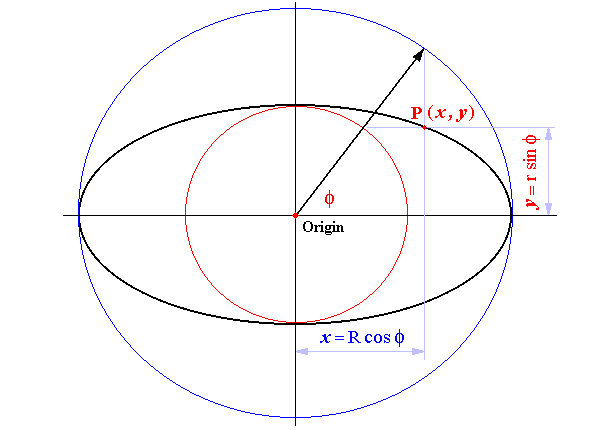
Parametric Equations of an Ellipse
R = Semi - Major Axis
r = Semi - Minor Axis
Differentiating with respect to f
dy/df = d(r · sinf)/df = r · cosf
dx/df = d(R · cosf)/df = R · (– sinf)
Since cosf = x/R and sinf = y/r
Substituting for sinf and cosf
dy/df = r · x/R
dx/df = R · (– y/r)
Slope at any point on the Ellipse = dy/dx
dy/dx = (dy/df) / (dx/df)
Substituting for dy/df and dx/df
dy/dx = (r · x/R) / (– R · y/r)
dy/dx = – (r ² · x) / (R ² · y)
Since R = Semi - Major Axis
and r = Semi - Minor Axis
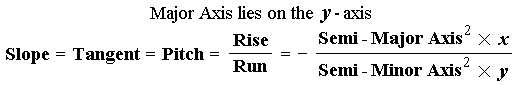
Formula for Slope of Ellipse at any Point
Formula for Slope of Ellipse at any Point
Explicit Differentiation

Resolving the ellipse 4x2 + y2 = 16 in terms of y explicitly as a function of x and differentiating with respect to x.

The relation may be written as two functions:

Differentiating the function in the upper two positive y quadrants:

dy/dx = d(16 - 4x2)1/2/dx

dy/dx = d(16 - 4x2)1/2/d(16 - 4x2) • d(16 - 4x2)/dx ... Chain Rule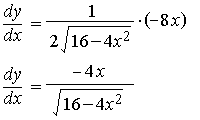



The solution for the function in the negative y quadrants returns the same terms but with opposite sign.
Implicit Differentiation

Using Implicit Differentiation to differentiate 4x2 + y2 = 16 with respect to x

d(4x2)/dx + d(y2)/dx = d(16)/dx

d(4x2)/dx + d(y2)/dy • dy/dx = d(16)/dx ... differentiating implicitly ... Chain Rule

8x + 2y • dy/dx = 0

2y • dy/dx = -8x

dy/dx = - 4x/y


Joe Bartok